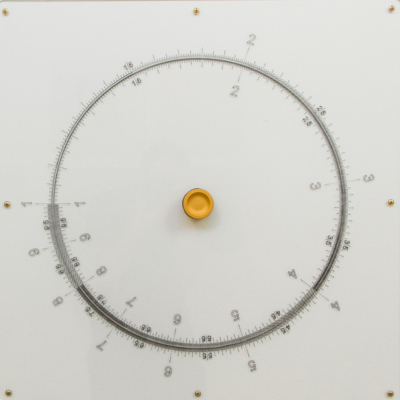
SLIDE RULE

Before the advent of electronic calculators, especially scientific ones, the slide rule was the only resource used by scientists and engineers in their daily calculations.

Invented by the English priest William Oughtred (1574-1660), this instrument had its improvement linked to several names: Richard Delamain (student of Oughtred) presents the circular slide rule (1630), R. Bissake presents the slide rule with sliding tongue (1654), J. Watt perfects the graduations of the scales (1779), Amedee Mannheim (French officer) introduces a movable cursor connecting the scales (1850), for example.

The main function of the slide rule is to perform multiplication operations, supported by the property log (ab) = log a + log b, transforming a product into an addition, on the logarithmic scale.

USEFUL LINKS

This Wikipedia page talks about slide rules, explaining their basic concepts and the operations that can be done using them, detailing each one of them. In addition, it brings some of the physical design, its history and comparisons with digital calculators.

This another Wikipedia page talks about the system, scales and theory behind slide rules. In addition, it presents some of the operations performed with it, through a table that explains how to obtain the result for each of them.                                                          

This Museu Virtual de Matemática's (Virtual Museum of Mathematics) page brings three very old slide rules: a simple and a circular one from 1622 and a cylindrical one from the 17th century. The interesting thing is to see pictures of the calculators that preceded the current calculators!

This SuperVPower's channel video explains how to use a simple slide rule, first introducing its elements and then showing how to obtain the results of multiplication, division, power and square root operations.                                                          

Finally, this SBEM's (Brazilian Society of Mathematics Education) article brings an analysis of how the circular slide rule can be used as a mediating instrument in the teaching of logarithms, with some activities that can be done with students.