KNOTS AND LINKS
Knot Theory is one of the most fascinating areas of Mathematics. Its origins date back to the late nineteenth century and it is currently part of the field called Algebraic Topology. It is striking that some concepts, proofs and open problems can be stated in simple language, allowing non mathematicians to have access to vast portions of the theory.
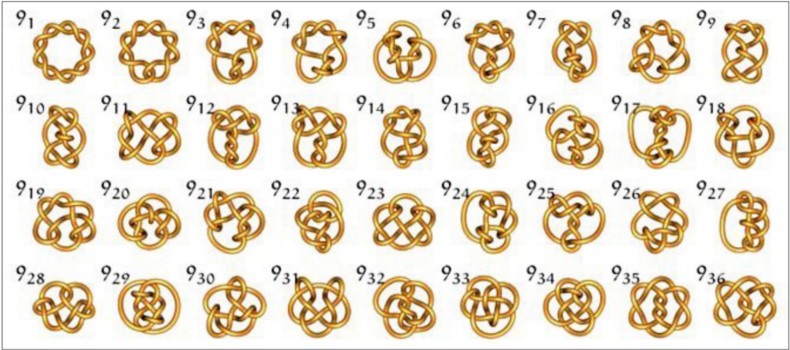
Image: A Knot Zoo.
Knot Theory studies closed curves in space without self-intersections. Two of these curves (or knots) are considered equivalent if one can be turned into the other by a continuous deformation. In the deformation process, self-intersections, ruptures or collapses (such as a knot so tight that it disappears) are not allowed.
One is usually not concerned with the exact shape of the curves. For example, any curve that can be deformed into a circle can be called a trivial knot.


Besides that, you can also relate some of the knots with its corresponding surfaces, which conects knots and link's study with surfaces topology's. This relation gets very clear in the next video!
If you want to know more about surface topology, check our page that talks about it!
