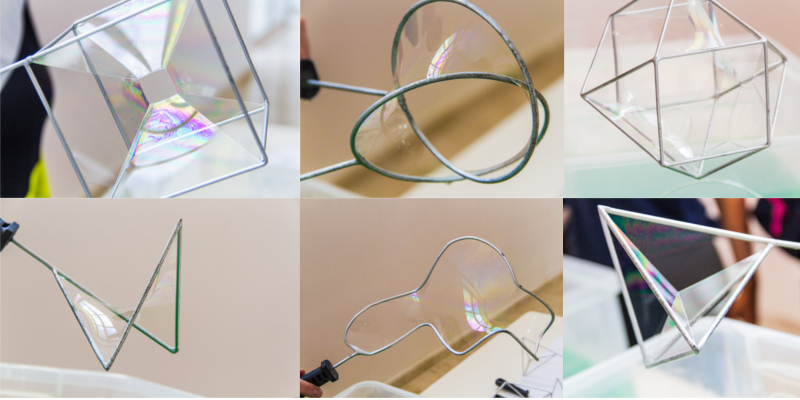
SOAP FILMS
Knowing the possible shapes that a soap film can take once its contours are already pre-defined (by metals) is what is called, in Mathematics, the Plateau problem.
When the film does not surround the air forming closed chambers, it has the property of minimizing area. This means that any nearby shape that has the same outline will necessarily have more area.
These shapes are therefore called minimal surfaces. Note, especially when the contours are edges of a polyhedron, that there are also internal edges created by the meeting of skins, which are sometimes even in planar form. This creates beautiful figures, sometimes more than one for the same outline.
The study of minimal surfaces is included in what is modernly called differential geometry.