CHLADNI PLATES

About 200 years ago, Ernst Florens Friedrich Chladni, from Saxony, experimented on vibrations of rectangular and circular metal plates whose center was kept fixed and with a free contour.

Image: Rodrigo Tetsuo Argenton.

He caused the vibrations with a violin bow and, in order to visualize the different modes of vibration of the plate, he pulverized it with fine sand, which ended up being deposited on the points of the plate that remained stationary during the vibrations (nodal points), forming beautiful figures (figures of Chladni) formed by special lines (the nodal lines).

Napoleon Bonaparte was so intrigued by this experiment that he offered 3,000 francs for the development of a satisfactory mathematical theory of plate vibrations. This award went to Sophie Germain, who modeled the problem with a partial differential equation of order 4. Later, G. R. Kirchhoff gave a more accurate treatment of the boundary conditions of the problem.

An approximate model to describe such vibrations is that of the vibrating membrane, for which the wave equation is used.

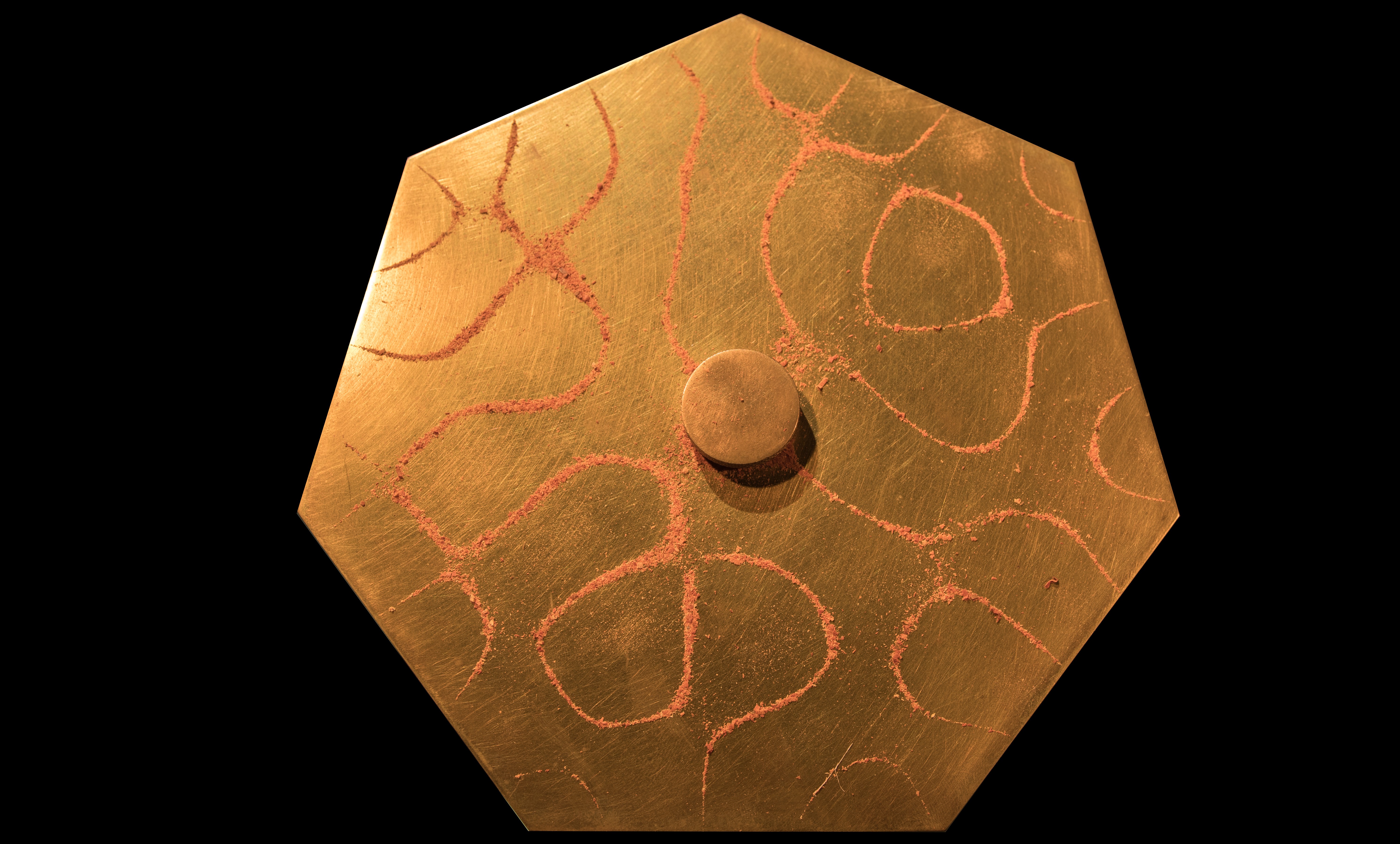
Chladni figure on a heptagon-shaped plate.
Image: Rodrigo Tetsuo Argenton.
                 
Formation of the figure of Chladni on a heptagon-shaped plate.
Video: Rodrigo Tetsuo Argenton.

USEFUL LINKS

This Wikipedia page talks about the formation of Chladni figures, bringing an analysis of what happens while they are being formed and the relationship with the study of waves and vibrations. The coolest thing is that the photos and videos on this page are from Matemateca's collection!

This Public Domain Review's article brings a summary of how it began - and how it was deepened - the study of the so-called Chladni figures. The page brings copies of the diagrams that Ernst Chladni himself used in his studies, showing a certain evolution of the drawings - and consequently of his work - on each page.

This AZEHEB Laboratórios Educacionais's channel video shows, by means of a device that generates independent frequencies, the formation of Chladni figures. Initially, you can see some slowly forming on a square plate. Then, other images appear formed on plates of different shapes, such as circular and triangular!

Finally, in this COMSOL's blog text one can understand why the Chladni plates have become something so important: they make it possible to visualize a sound, something that we think is even contradictory. In addition, the page gives a summary of how image formation works and also shows a computer simulation of the experiment.