HARMONIC SERIES

Is it possible to stack equal plates, without using glue, so that the length of the projection of the set on the table is arbitrarily large?

The fact that the harmonic series 1 + 1/2 + 1/3 + 1/4 + … diverging to infinity guarantees the affirmative answer to this question. Just stack the plates like this: for each plate, the set of plates above it has the center of mass at its end, that is, it is in the limit position to fall. By the way, if you want to know more about center of mass, take a look at our exhibit that talks about it!

Image: Alisson Ricardo.

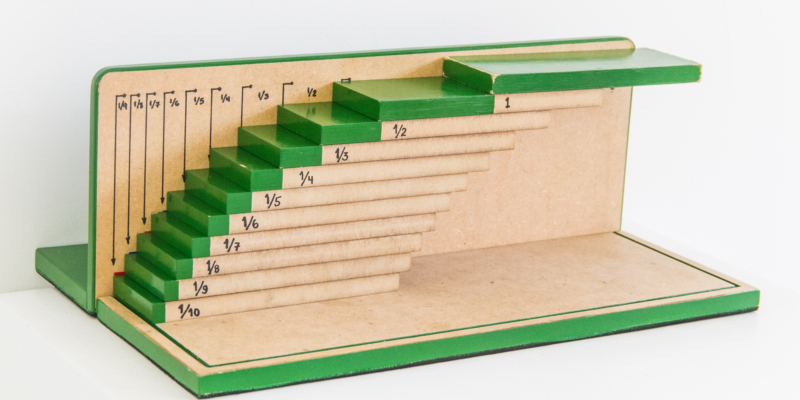
Although the harmonic series tends to infinity, this happens very slowly. If, since the Big Bang, approximately 14 billion years ago, a new term of the series had been added every second, this number would still not have exceeded 50. This is what we mean by very slowly!!!!

This idea becomes clearer by observing some partials of the harmonic series:
1 + 1/2 + 1/3 + ... + 1/10 = 2,92…
1 + 1/2 + 1/3 + ... + 1/100 = 5,18…
1 + 1/2 + 1/3 + ... + 1/10000 = 9,78…
1 + 1/2 + 1/3 + ... + 1/1000000 = 14,39…

USEFUL LINKS

This Wikipedia page talks about mathematical series, giving their definition and classification in terms of convergence. It also talks about convergence and divergence of the series, in addition to presenting some important types, such as the harmonic. In addition, it talks about functions defined by series and generalizations in normed spaces.

This another Wikipedia page speaks specifically about the harmonic series, which are studied by the piece presented here. On the page, comments are made on the sum of reciprocal primes, on alternating harmonic series, in addition to explaining the divergence of this type of series.

It was seen that the harmonic series is divergent, a concept explained in this Wikipedia page. In it, its precise definition is given and the sum method is presented, explaining some of its properties. Finally, we talk about the Abelian mean and the Abel sum.

And if you are not convinced that the harmonic series is divergent, this Khan Academy's video demonstrates this important, though not so obvious, property of harmonic series, through algebraic manipulations and direct comparisons.