POLYHEDRA

Kepler studied polyhedra a lot. His fascination was so great that he tried to explain the distances of the planets from the Sun on the basis of a scheme of spheres and polyhedra inscribed on each other, in his book Mysterium Cosmographicum.

Interest in polyhedrons dates back to Ancient Greece and continues to this day. To date, there is research being done on polyhedra and also polytopes, which are their generalization to higher dimensions and to non-Euclidean geometries, such as hyperbolic or spherical.

In addition to aesthetic pleasure, polyhedra provided beautiful, simple and surprising theorems. After the Renaissance, we highlight Euler's Theorem, relating the number of faces, edges and vertices of a polyhedron by the formula V+F=A+2.

Euler's formula is valid as long as the polyhedron is equivalent, in the topological sense, to a sphere. It means, intuitively, that we can “inflate” the polyhedron until it becomes a sphere.

For polyhedra of this type, Descartes' Formula is also valid, which states that the sum of the angular deficiencies of the vertices is always equal to 4π (or 720 degrees). The angular deficiency of a vertex is how far it takes for the sum of face angles incident on that vertex to reach 2π (or 360 degrees).

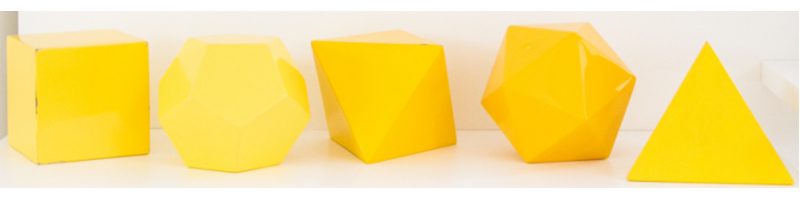
CONVEX POLYHEDRA WITH REGULAR FACES

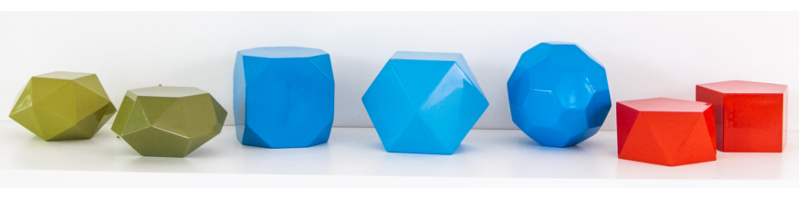
UNIFORM POLYHEDRA Uniform polyhedra are those in which all vertices are indistinguishable from each other. One can say that 'what you see of the polyhedron from a vertex is exactly what you see from any other vertex'. They are classified into 'platonic', 'prisms', 'antiprisms', and 'archimedean'.


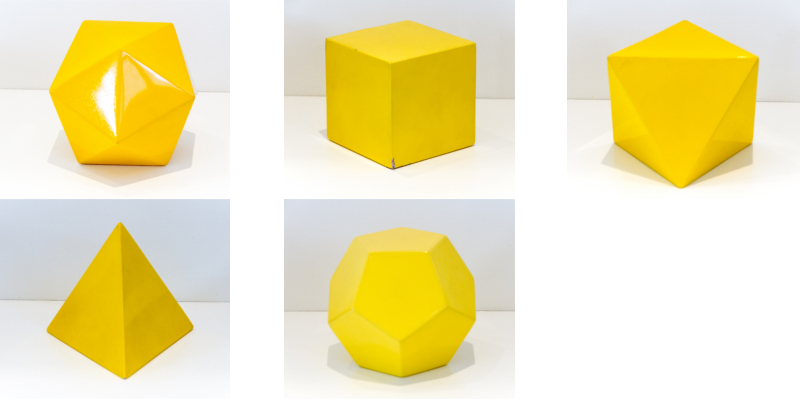
PLATONIC These are the uniform polyhedra with equal faces (tetrahedron, cube, octahedron, dodecahedron and icosahedron).

PRISMS Parallel bases connected by squares (infinite family).
ANTIPRISMS Parallel bases connected by triangles (infinite family).


ARCHIMEDEANS All other uniform polyhedra (finitely many).


NON-UNIFORMS Pyramids, domes, deltahedrons, cuts of uniforms etc. They can be both elementary and composite - composite polyhedra are those which may be split by a plane into two other convex polyhedra with regular faces, while the elementary ones can't. They are finitely many.

If you want to know more about these amazing subject, watch the two videos above! In the video "Plato's Polyhedrons: Dual and Inscribed, Profesor Doctor Lucia Satie Ikemoto Murakami mentions some plans, which are available on the Symmetries page. Besides, seize the opportunity to check the other cool videos in the Matemateca's channel on YouTube!

USEFUL LINKS

This Wikipedia page brings a very complete explanation of polyhedra, starting with the definition and a more general view. It also specifically talks about different types of polyhedra, such as regular, non-regular and convex, as well as mentioning some other important families. It talks about transformation operations on solids, among other details on this embracing topic.

Thinking specifically of Platonic solids, this Wikipedia page provides a very complete overview of the topic. It talks about Cartesian coordinates, combinatorial, geometric and metric properties, classifications - both by geometric and topological proof -, symmetries and operations on Platonic solids. In addition, it mentions a visualization in nature, through liquid crystals with solid symmetries!

Euler's Theorem represented a great advance in the study of polyhedra and geometry. This Institute of Mathematics, Statistics and Scientific Computing at Unicamp's page talks about Leonhard Euler and his theorem applied to convex polyhedra, presenting an induction proof of its validity in this case. Also, it talks about how his theorem is not valid for any polyhedron.

If the condition of Euler's formula on the polyhedron being topologically equivalent to a sphere was not clear, this Wikipedia page will be ideal in your studies (or curiosities). Topology is a branch of mathematics that studies topological spaces, divided into some branches, such as geral and the algebric. On this page, you can understand a little about its history and elementary aspects.

Johannes Kepler, an astronomer fascinated by polyhedra, wrote the book Mysterium Cosmographicum, in an attempt to explain the distances of planets from the Sun. This Wikipedia page gives an overview of what is covered in the book, including its theological and philosophical basis and the philosophy and epistemology of the sciences more generally.                                                                                       

Finally, polytopes are the generalization of polyhedra to higher dimensions. This Wikipedia page talks more specifically about convex polytopes, giving their definition, some examples and properties, such as topological and simplicial decomposition. It also presents some algorithmic problems for a convex polytope and the construction of its representations.