MATHEMATICAL TILING

The art of mathematical tiling consists of filling the plane with polygons, without overlaps or holes. This technique is used in a wide variety of applications: wallpaper, decorative floors with ceramics or stones, wooden floors and ceilings, fabric printing, knitting and crochet, in the packaging or stacking of similar objects, etc.

Image: Gastão Guedes.
Image: Alisson Ricardo.

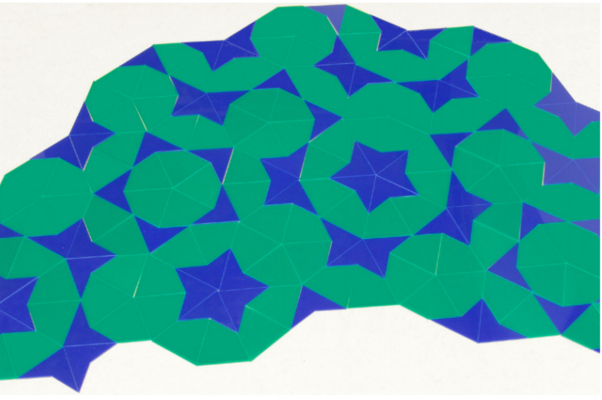
There are only 11 types of mathematical tiling that use only regular polygons and keep the same distribution of tiles at each vertex. However, none of them allow the use of regular pentagons. With a single type of polygon, we have the tiling of equilateral triangles, squares and hexagons, but also with irregular pentagons. Furthermore, it is not difficult to see that any quadrilateral is used to tile the plane.

Penrose mosaics use two special polygons, the “parrot” and the “glider”, to form non-repeating tiles.

Image: Alisson Ricardo.

USEFUL LINKS

This Wikipedia page talks about mathematical tiling (also called tessellation), tells a little about its history and presents its use in mathematics, including its application in dimensions greater than 2 and even even in non-Euclidean geometry. This other Wikipedia page brings more specifically the tiling with convex regular polygons.

This summary found in Pi.math page shows the presence of mathematical tiling in everyday life, often in places where we don't even think about mathematics. In addition to the photos, a mathematical definition and an explanation of how to check if two tiles are congruent with each other are also provided.                                                        

This UNB's (Brasília's University) mosaics presentation brings a more formal definition of what tiling is, from the union of polygonal regions, and also some interesting conclusions on the subject. In addition, it brings several examples and images to make the theme tangible.                                                                                                                

This Portal do Professor page presents a class involving tiling, presenting the theme from a deeper study of the geometry involved in it. For example, the page makes careful consideration of necessary and sufficient conditions for polygons to fit together and also some analysis of polygon congruence.

Finally, if you are interested in the topic and would like to try to assemble your own tiles, this Geogebra's resource lets you choose some of the polygons (triangles, quadrilaterals, pentagons, hexagons and other more general polygons) and start tiling! Through the interface, it is still possible to connect the polygons by their vertices.