GEARS

Start the movement with all arrows vertical and pointing up. Start spinning by looking at the blue gear. How many turns of the blue wheel does it take for the mechanism to return to the starting position?

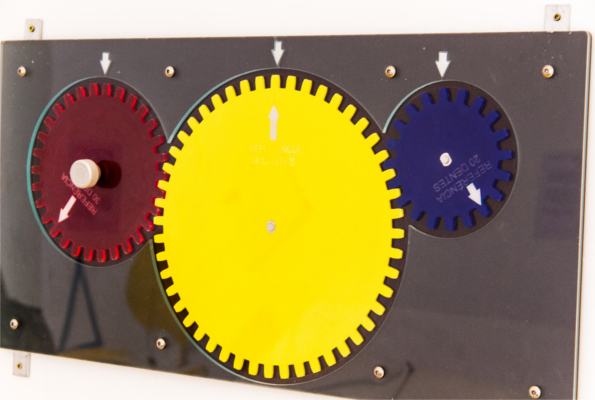
You can answer the challenge by simply counting the turns of the blue gear. But he could also predict the outcome without even putting his hand on the apparatus.

What matters here is the number of teeth on the gears. The arrow on the blue wheel returns to its initial position when this wheel makes one revolution, that is, when 20 gear teeth have passed. Again the arrow returns to the starting position when 40 teeth, 60 teeth, etc. have passed. Something similar happens with the other wheels.

Conclusion: all arrows will be back in the starting position when the number of teeth that pass through is a multiple of 20, 30 and 48 at the same time. The first time it occurs it is the least common multiple (LCM) of the three numbers. So, how many turns does the blue wheel make for the mechanism to return to its initial position?

Image: ENEM (National High School Exam) 2017.

The sprocket principle is used on bicycles, so the number of pedal strokes need not be exactly equal to the number of turns of the wheel.

If you want to see a little more about this mechanism, check out the video below! Enjoy and check out the other cool videos on Matemateca's channel on YouTube!

                 

USEFUL LINKS

This Wikipedia page brings a general explanation of what gears are, the bases of this piece. It shows the main types of gears, such as conical and helical, and some calculations involved in their analysis, in addition to a photo gallery.

This Marcelo Pereira's channel video is a class on the principle of cog wheels. This principle is established with the help of a tool called Solidworks, useful in creating models and three-dimensional sets, facilitating, for example, mechanical projects.

The concept analyzed with this piece is the least common multiple, explained in this Wikipedia page. In it, the exact definition and a way to calculate efficiently are given. It also brings an alternative method and some properties related to the theme, in addition to presenting concepts that are also important in this study, such as greatest common divisor.                                                                                                                      

Finally, the mathematical use of gears is not limited to calculating the least common multiple. Blaise Pascal, a French mathematician, already used them in the construction of his calculator, known as Pascalina (or Pascal's Machine). In this Faculdade de Ciências da Universidade de Lisboa page, the operation and history of such a mechanism are explained, in addition to bringing other machines as an example for comparison.